“If you have ever studied geometry, you remember that by a course of reasoning, Euclid proves that all the angles in a triangle are equal to two right angles. Euclid has shown you how to work it out. Now, if you undertake to disprove that proposition, and to show that it is erroneous, would you prove it to be false by calling Euclid a liar?” Political Debates Between Lincoln and Judge Douglas. Fourth Joint Debate at Charleston, 1858.
Where to begin
Hopefully, you found our journey through the number line interesting. Perhaps you learned a thing or two, or at least now view the number line from a different perspective. But let’s be honest, you knew a lot about a number line before you started reading Lazarus Math. Although there are a lot more treasures we could uncover on a simple number line, I’d rather unveil less straight things in our limited time together. Let’s jump right into round things, like curves and circles.
I suggested in the previous section to view a circle as a “number circle,” or a type of “round number line.” Have you ever thought about a circle as a number line, similar to our straight number line, except round, perfectly round? That may be a foreign concept but now that we have GPS technology, we’re familiar with measuring distances on paths that are not straight. We need to upgrade our math mentality to think of curves as things we can measure even though they aren’t straight.
But how do we measure round things? Sure, we can measure round things with our GPS technology, but how is it done? Let’s start at the beginning with the Ancient Greeks and Archimedes. Archimedes certainly didn’t have a smartphone, yet he pioneered the idea of measuring round things by simply using some clever ideas, ideas that you and I potentially could have thought of had we taken the time.
Almost everything we will explore in Lazarus Math is somehow related to a circle. It’s not that our goal is to study a circle. Our goal is to do fun and interesting math, so we journey on that path. However, inevitably, we return to a circle. Circles are like math magnets. We just can’t get away from them. As the saying goes, if you can’t beat ‘em, join ‘em.
How would you do it?
Lazarus Math is about lighting up your imagination, so imagine. How would you measure the distance around a circle? You may remember the formula from high school, but let’s not use a formula here. Let’s create one.
Where do you begin? Where do Mozart and Beethoven begin? Where do Shakespeare and Hemingway begin? We might think that they started with a blank sheet but, in reality, they would have started with what they knew. So let's start with what we know and be willing to wander from there.
What do we know? We know straight things. How can we use straight things to measure round things? Like we do with many large projects. We break them down into small tasks. Is there anything “straight” on a circle?
Like clockwork
The circle is perfectly symmetrical, so locating the center seems like a natural thing to do. Once we identify the center, then we can think about the points on the circle in relation to the center. By definition, the distance to any point on the circle from the center is the same. Recall we give it the fancy name radius, or as a nickname. We don’t care what circle we’re exploring, but we want to simplify whenever possible. Let’s set since that seems to be as simple a circle as we can create.
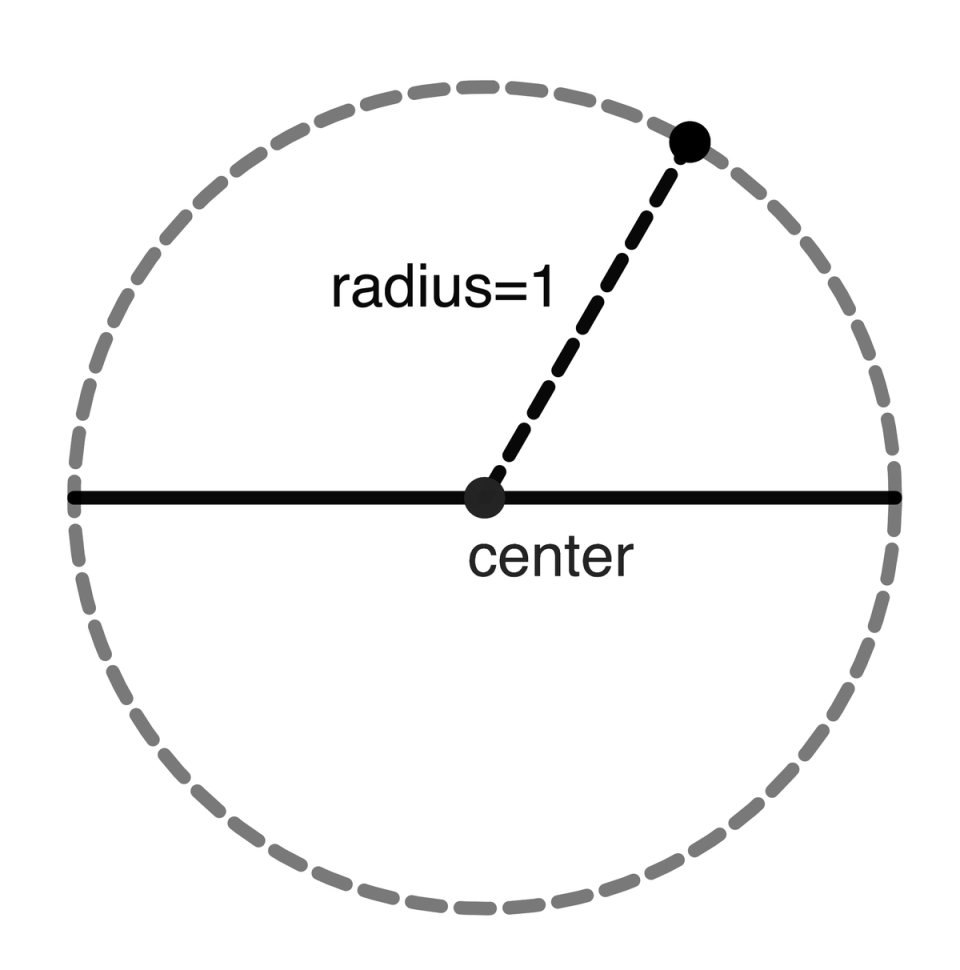
Figure 1. A circle with radius 1
If we do a quick inventory of what we have accomplished, we created a circle, found a center, and declared the distance from the center to any point as distance 1.
One thing I think about if I’m contemplating the distance around a circle is a clock. A clock is something we know a little bit about. What if we organize our circle like a clock? That means we could draw a radius for where the numbers are on a clock as a way to “divide and conquer.” There are 12 numbers on a clock, so we could start with 12. But that seems like a big jump, so let’s try 6. We want to preserve symmetry so we can choose either the even numbers or the odd. Let’s choose the odd numbers. Good, now take a step back and review our work.
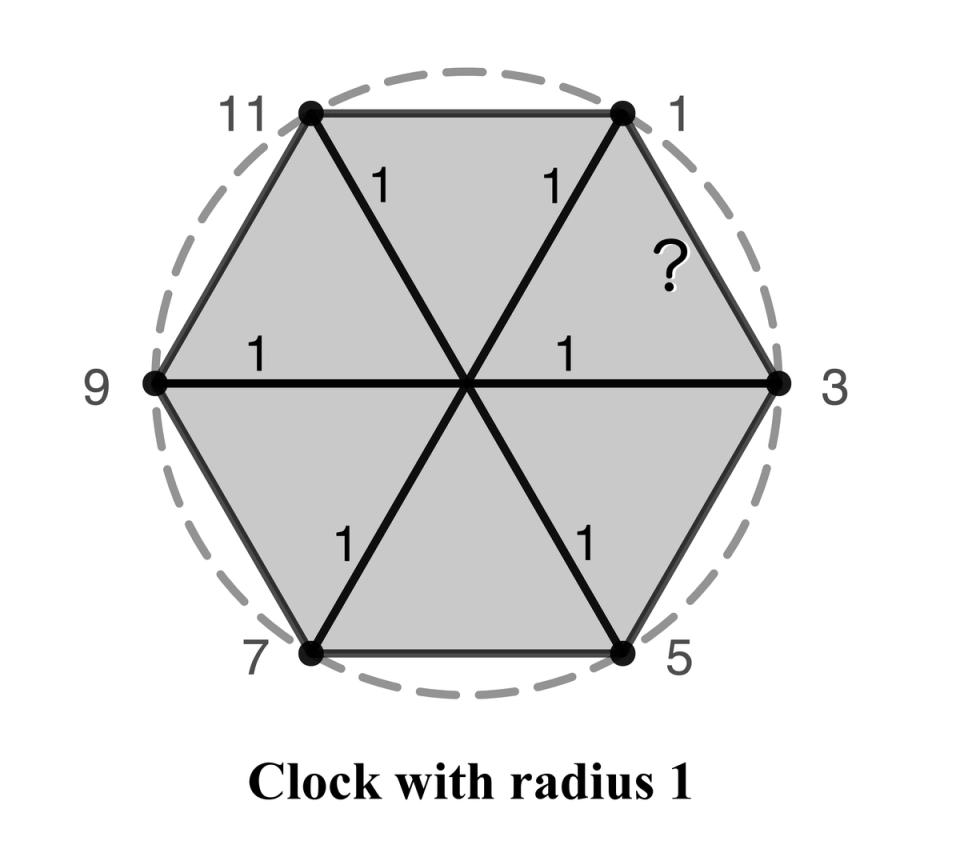
Figure 2. Circle organized into 6 equal parts like a clock
What do you notice? From Figure 2, does it appear that the distance from the center to the circle is the same as the distance between our clock numbers? We declared the length of our radius as 1. Can we declare that the shortest distance from 1 o’clock to 3 o’clock is also 1?
Symmetry from 6
If you recall, there are 360 degrees in a circle. Since we divided the circle into 6 equal parts, we know there are 60 degrees in each part. Notice each part makes a triangle. Let’s examine one triangle. Recall a triangle has a total of 180 degrees and we just used 60 degrees. Thus, there are 120 degrees remaining for the other two angles.
Once again, symmetry is our friend. With the two radii giving us symmetry in the triangle, then we know the angles for the other two sides must be the same. That means each of the remaining angles is or 60 degrees.
As a result, our triangle is a 60/60/60 triangle. This means the triangle is perfectly symmetrical. Because it has perfect symmetry, all the angles have equal measures, and the sides must also have equal lengths. We are now confident all sides must equal 1.
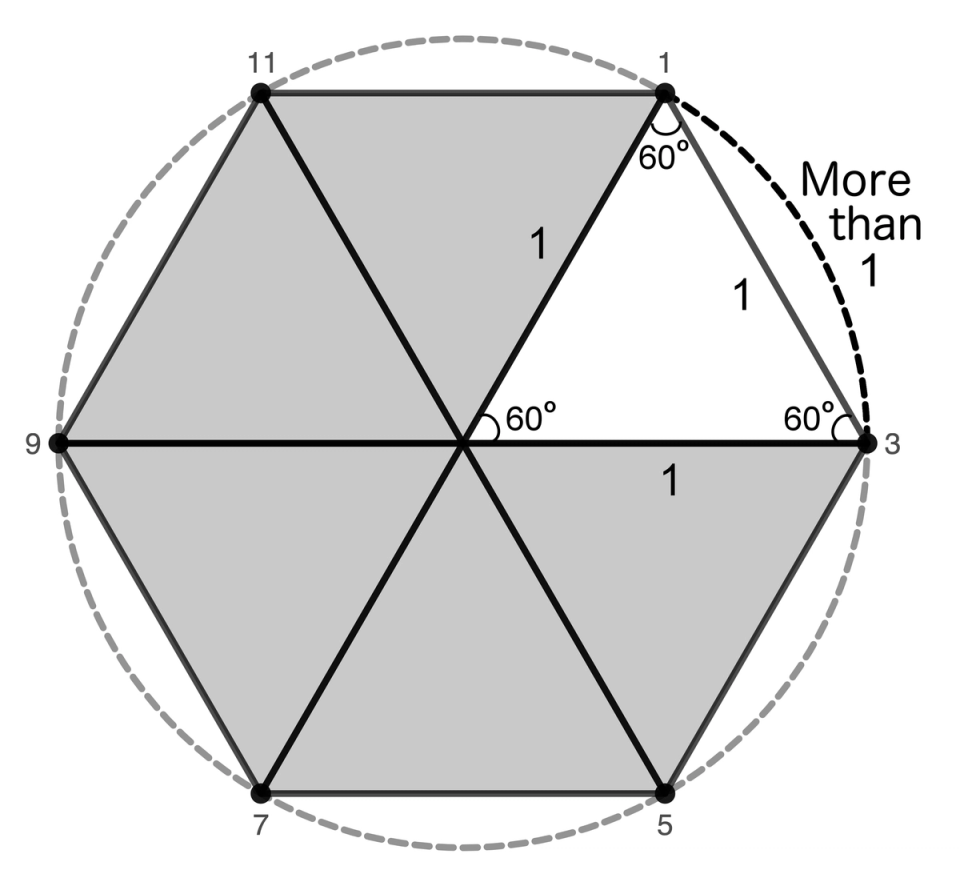
Figure 3. Six triangles, each with the same angle measurement
How does this help with our goal of measuring the area around the circle?
First estimate
We have shown that the length of the side from 1 o’clock to 3 o’clock is 1. We have 6 of these sides, all with the same length as we go clockwise. Thus, consider the distance around our clock from 1 o’clock to 3 o’clock to 5 o’clock and all the way back to 1 o’clock. If we travel along the path of the straight lines, which is the part of the triangles, we know the distance is or 6.
Compare the distance around the circle to the distance around the perimeter of our 6 triangles. We know the shortest distance between any two points is a straight line (at least in the geometry we’re studying here). The circle and our 6 triangles share the same points but, in every case, the distance to travel around the circle is more than the distance along the straight line.
Thus, we can conclude that the distance around the circle is more than the distance along our triangle perimeter. Therefore, the distance around the circle must exceed 6.
That wasn’t too complicated was it? It didn’t require fancy formulas or heavy math. Just some thoughtful questions. We don’t know the exact distance, but we know it is more than 6. That is not a bad estimate given how easy it was to derive. Like we did in Buffon’s problem, we practiced the skill of approximating. Sometimes our approximate method actually is on the correct path to calculating the result exactly. If that is true here, that leads to the question: how can we remain on this path yet improve our estimate?
Next estimate
Our 6-sided shape is called a hexagon. You may recall the general name for these shapes is a polygon. A polygon needs at least 3 sides, so a hexagon qualifies as a polygon.
Our goal is to “stretch out” our polygon so that it approaches the circle. How do we accomplish that agenda? Consider the result if we divide our circle into finer pieces. For example, we could divide the circle into 12 pieces rather than 6. Now we have created a shape that resembles a clock using all 12 numbers. Here is a picture with our circle approximated by a 12-sided polygon, which contains 12 equivalent triangles rather than 6.
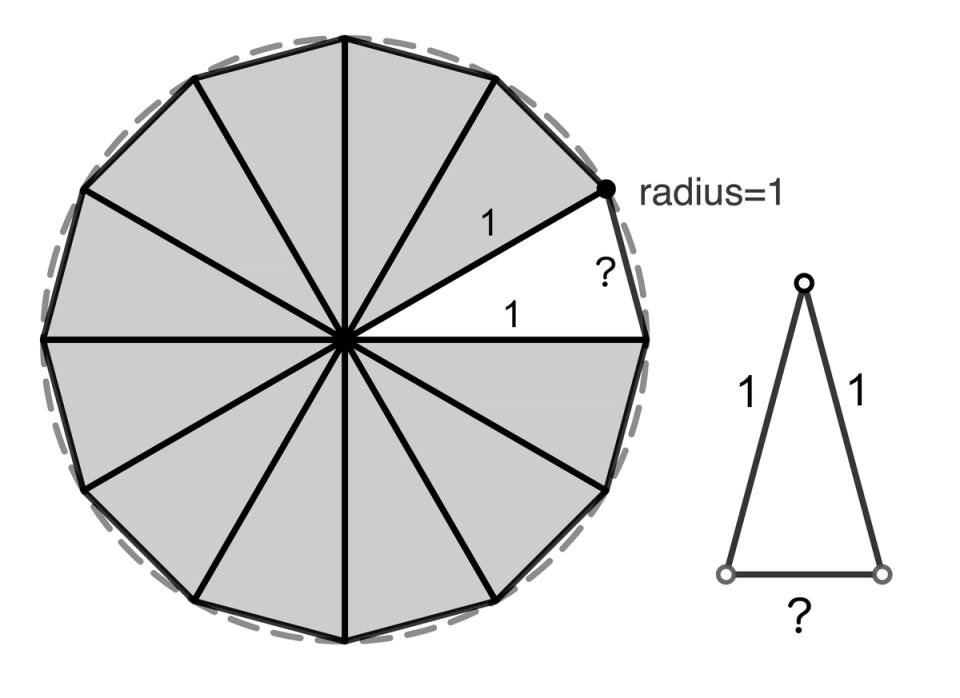
Figure 4. Circle approximated by 12 triangles
I extracted one of the triangles and set it flat so we can view it in detail. Recognize this is just one of 12 triangles where each triangle has the exact same properties.
Notice the perimeter of our polygon is approaching the circle, even though all the edges are straight. If we can measure the length of one side, then we only need to multiply it by 12 to calculate the entire perimeter since we’ve maintained symmetry.
How do we measure the length of one side?
There are several options, depending on how much old school vs. new school you want to pursue. If this was a tangible circle, like a pizza, you could cut it into 12 equal pieces and measure the distance from point to point along the crust.
But we prefer to be in our imaginary world where everything is clean. Let’s review one of the triangles. From symmetry, the measure of one angle is now or 30 degrees. That means we have or 150 degrees to allocate to the other two angles of the triangle. Again, because of symmetry, we can split the 150 decrees into two angles of 75 degrees. Thus, the triangle is a 30/75/75 triangle. We want to measure the length of the short side. Notice each of the other two sides is a radius of the circle, so their lengths are 1.
That certainly helps our cause, but the path remains fuzzy. You may recall from trigonometry that right triangles have special properties that make it easy to calculate the length of sides. We don’t have a right triangle. Yet again, symmetry comes to our rescue. We can cut our triangle in half and split our 30-degree angle evenly to create two new triangles with 15-degree angles. We know the other angles didn’t change, so each remains 75 degrees. That means the new angle we created is or 90 degrees. We created two right triangles!
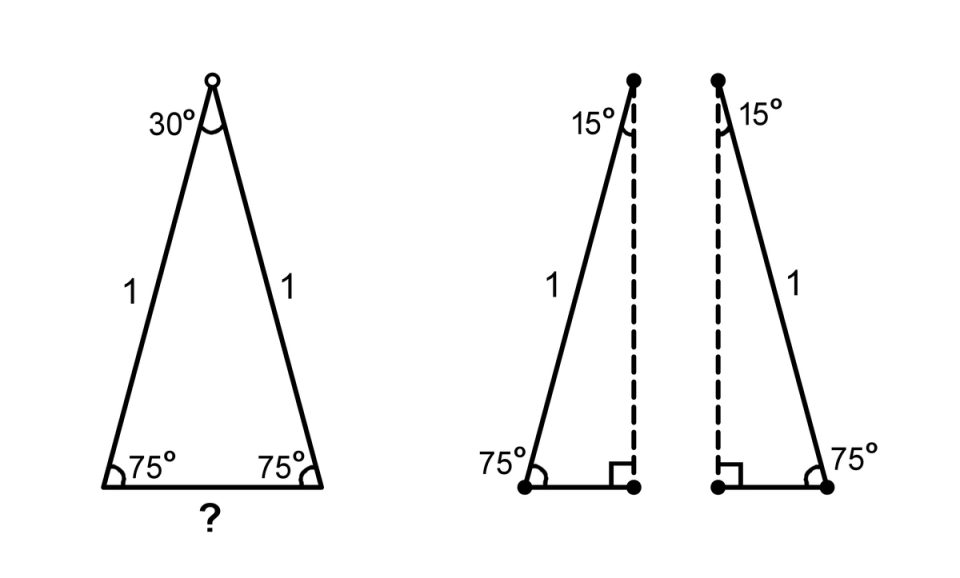
Figure 5. Creating two right triangles from one triangle
As a result, we have a right triangle, and we need the length of its base. We have options here. Our inventory of what we know tells us the length of the side opposite our right angle (which we call the hypotenuse) is 1. The reason we created the right angle was so we can use a handy trick you likely learned in high school. Remember the sine and cosine? The details may be fuzzy, but perhaps you remember that a non-hypotenuse side of a right triangle divided by the hypotenuse is either the sine of the angle or the cosine of the angle. That means we can solve for the missing side by using either the sine or cosine function. Let’s use the sine function, which is opposite / hypotenuse. The angle opposite the side we need is 15 degrees. That means the sine of angle 15 equals the length of the opposite side. To solve this, one option is to go the easy way and use our calculator to find the sine of 15. We’re taking the easy route this time and using our calculator to get 0.2588.
Because we cut our original triangle in two, 0.2588 is half the length of the side of our original triangle. Recall we have 12 triangles. That means the length all the way around the polygon is , which is 6.212.
Notice we identified 12 equally spaced points on the circle, similar to the numbers on a clock. We measured the shortest distance between points, which summed to 6.212. This estimate exceeds our previous estimate of 6 because we refined the estimate even more by doubling the number of triangles.
We know this is still an estimate because we are measuring the distance around using straight lines rather than the circle. We know this estimate still underestimates the true distance around the circle as we are still measuring the shortest distance between 12 points using straight lines.
Again, it appears we’re on the correct path, so we can repeat the process. We could repeat by creating another polygon with twice the number of sides, which increases from 12 to 24. But let’s try a faster pace and use the clock as our guide. Rather than using the 12-hour numbers, let's divide the clock by minutes to get a better approximation to a circle.
Better estimate
Now, try to quickly calculate the estimate based on what we’ve observed so far. There are 60 minutes, so we create a polygon with 60 sides, equally spaced. That produces 60 triangles, all copies of each other. We want the angle connected to the center of the circle. Next, divide 360 degrees equally into 60 triangles. This produces an angle of 6 degrees for our triangle of interest.
But this isn’t a right triangle. We can fix that problem. Like before, create a right triangle by splitting this triangle in two to create a triangle with angle 3. Then, this is another right triangle, and we can calculate the length of the unknown side as sine 3. Next, multiply by 120 since there are of these sides. The result is 6.280.
Even though we are creating a lot of triangles, our estimates are not changing as much. That indicates we’re approaching our answer.
Our final answer is this. The distance around the circle is the mystery number times the distance across. The distance across is 2. Therefore, the correct answer is 2 × , which is equal to 6.283 to 3 decimal places. The difference between the actual distance and our latest estimate is to 3 decimal place accuracy.
Notice we are estimating the mysterious by using triangles. With 60 triangles inside the circle, our best estimate for so far is or 3.140, which is . If we recognize the pattern, we can cut our angle in half again and double our count from 60 to 120, which creates the estimate . We’re nearly there as the correct answer to 4 decimal places is 3.1416. The difference between actual and our estimate is to 4 decimal places.
Perspective
The strategy we used is similar to how Archimedes estimated . He started with a six-sided polygon like we did and continued to divide the polygon until he had 96 sides. However, unlike us, he didn’t use the calculator and the sine function. We clearly took a modern approach. There are sophisticated ways to estimate the sides without a calculator if you desire to follow the pure Archimedes approach, but it is labor-intensive. There were other techniques Archimedes used that we glossed over, but this example highlights his approach.
The main thing is to uncover the idea of measuring something round by taking something straight and organizing it in ways that approach the round shape. What Archimedes accomplished was a big deal in the history of math. He knew that the ratio of the distance around divided by the distance across a circle was a constant, but he didn’t know what that constant was. This pursuit of estimating and being able to measure something round was a landmark change in the history of math.
Let's test how well you understand the measurement around a circle. We'll consider measuring the distance around a sphere. Notice a sphere has one more dimension than a circle. However, if we measure the distance around a sphere, we're essentially creating a cross-section of a sphere which is a circle. We'll simply measure the distance around the sphere using a string. Now measure the distance around these three objects: a golf ball, a basketball, and the earth (yes, we need a lot of string). Of course, we'll assume that each of these objects are perfect spheres. If it helps, you can picture 3 strings that represent the distance around each of these perfect spheres. Now, assume that the radius for each of these spheres increases by one inch. This one-inch radius increase would be quite evident on the golf ball but would be virtually unnoticed for the much larger earth. For each of these enlarged spheres, remeasure the distance around. Then, compare the length of the string for the enlarged object to the original object. Our question is: which string increased more in length?
My intuition is that the string used to measure the distance around the earth increased much more than the other two. Clearly, it requires a large string to wrap around the earth. If the radius for the earth increased even a small amount, such as one inch, my intuition would be that the change in the length of the string would be much greater for the earth than for the golf ball. However, my intuition is wrong because the string will increase the same amount for each sphere. This is easy to prove.
Recall the distance around a sphere is 2 × × where is the length of the radius. Notice if we increase the radius by 1, then we increase the distance by 2 × regardless of the initial value for the radius. This leads to the not so intuitive answer that the distance increases the same for all spheres when we increase the radius by the same one inch.
One more take-away from this example is how we measure angles. For this story, we used the definition that the distance around a circle is 360 degrees. In high school, you were likely taught that there is a way to measure angles other than using degrees. This other way is using radians. Of course, the measuring tool we use doesn’t change what we are measuring, assuming we use those tools accurately. You can measure the length of your kitchen using a yard stick or a 12” ruler. Whether we measure the distance in yards or feet, the length of the kitchen doesn’t change.
Even if you are more comfortable using 360 degrees, I will often use the radian measure for the remainder of Lazarus Math. Why use the radian measure when we already “know” 360 degrees?
It’s kind of the same reason you likely use your finger to navigate your smartphone. Because it is the natural thing to do. Sure, you could get a device, like a stylus, but that device gets in the way of what is the “natural device,” your finger. Using radians is the “natural way” to measure angles. I will explain why it is more natural when we start using radians later in Lazarus Math.
Now that we’ve identified how to measure the distance around a circle, let’s try something that may appear more difficult: calculating the area within a circle. This is our next target.