Revisiting the Means

“He has made everything beautiful in its time. Also, he has put eternity into man's heart, yet so that he cannot find out what God has done from the beginning to the end.” Ecclesiastes 3:11

Revisiting the circle

Before we finish our journey, let’s play with an interesting problem that will connect several ideas we have seen. We just learned about how Kepler identified that an ellipse is the key to how planets orbit around the sun. In the previous section, we explored how the geometric mean and arithmetic mean are hidden in a circle. There are many beautiful connections between a circle and an ellipse. You may recall it required considerable effort to identify how the geometric mean and arithmetic mean are embedded in a circle. Both means also exist in the ellipse but are easier to identify. For example, we identified that the geometric mean of 2 and 8 is 4 and the arithmetic mean of 2 and 8 is 5. As a reminder, here is the circle that illustrates these two means.

I removed much of the detail we had previously identified in this circle. I only presented the key components, which are the two numbers (2 and 8) and their two means (4 and 5). Previously in our field goal problem, we identified the number 8 as the distance from point A to Z. Because of the symmetry of a circle, we can also identify the number 8 as the distance from point B to point C, where C is the intersection of the tangent line at the top of the circle and the line through A and B. We know the diameter of the circle is 10, so the distance from the bottom of a circle to the top is 10, which is the arithmetic mean times 2. I changed our definition for distance 8 to be consistent with how we will define 8 in an ellipse.

The ellipse

How does an ellipse illustrate the same two means using the same two numbers? The easiest way is to consider an ellipse centered at the origin. Let’s assume the widest part of the ellipse is along the $x$-axis. The widest part of an ellipse is called the major axis, and the narrowest part of an ellipse is called the minor axis. The two points on the ellipse that also lie on the major axis are called the vertices. Thus, the length of the major axis of an ellipse is the distance from one vertex to the other. Since the longer part of the ellipse is along the horizontal, the shorter part of the ellipse is the height. If we have this setup, then the general formula for an ellipse is $\frac{x^2}{a^2}$ + $\frac{y^2}{g^2}$ = 1, where $a$ is the arithmetic mean embedded in the ellipse and $g$ is the geometric mean embedded in the ellipse. Since we want an arithmetic mean of 5 and a geometric mean of 4, then we can write the equation for the ellipse as $\frac{x^2}{5^2}$ + $\frac{y^2}{4^2}$ = 1. In short, we can easily identify the arithmetic and geometric means from the formula of an ellipse.

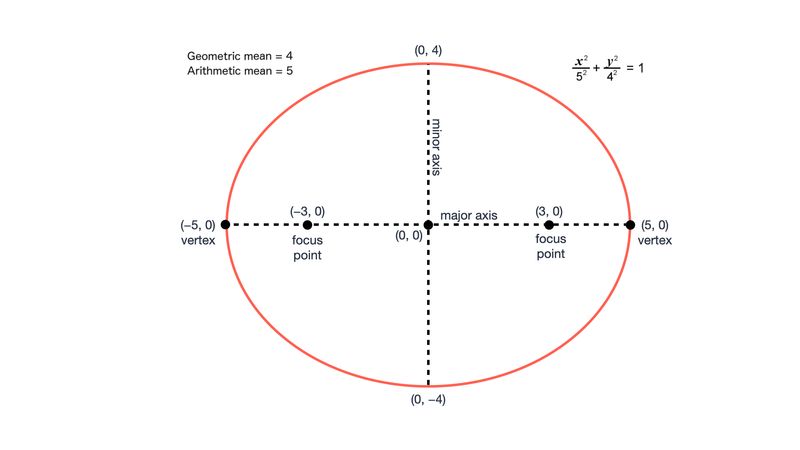
We explored this concept in great detail for a circle when we reviewed our field goal problem. Let’s visualize the two means on an ellipse using the ellipse for $\frac{x^2}{5^2}$ + $\frac{y^2}{4^2}$ = 1. Simply start at the center of the ellipse and travel along the major axis until you reach a point on the ellipse. The distance from the center of the ellipse to this point is 5, the arithmetic mean. Likewise, start at the origin and travel along the minor axis until you reach a point on the ellipse. The distance traveled is 4, the geometric mean.

The two means are clearly evident in the ellipse, but that is like giving the answer without stating the question. We haven’t identified how to determine the two numbers we are calculating the means for, which in our example are 2 and 8. In order to identify these two numbers, we need to locate the two focus points (or foci).

The foci

One way we can identify the two foci is to use the equation $f^2 = a^2 - g^2$, where $a$ and $g$ are the same means previously defined and $f$ is the distance from the center of the ellipse to each focus. Thus, we get $f^2 = 5^2 - 4^2 = 25-16=9.$ As a result, $f=3$, which is the distance from the origin to the two foci $(3,0)$ and $(-3,0)$. Next, let's identify the two numbers, 2 and 8, that we are calculating the arithmetic and geometric means for. Simply take the arithmetic mean, 5, and add and subtract the distance from the origin to one of the foci, which is 3. Thus, the two numbers are $5+3=8$ and $5-3=2$.

We can write this in a formula as $a+f$ and $a-f$, where $a$ is the arithmetic mean and $f$ is the solution to the previous equation. We can also visualize these two numbers on the ellipse. Travel along the major axis from one vertex to the other vertex. If we’re going left to right, that indicates we’re traveling from the point $(-5,0)$ to $(5,0)$. The total distance is 10, but we want to divide this journey into two parts. We stop at the first focus and record the distance traveled, which is 2. Then travel to the other vertex and note the distance traveled is 8. In other words, the distance from a vertex to a focus is either 2 or 8.

However, the two foci are not just interesting points that allow us to calculate these two numbers. Rather, these two points are the starting points for drawing an ellipse. What makes foci special is the sum of the distances from each focus to any point on the ellipse is the same. We know one point on this ellipse is $(5,0)$. The distance from $(-3,0)$ to $(5,0)$ is 8 and the distance from $(3,0)$ to $(5,0)$ is 2. Thus, the common distance is $8+2$ or 10. As a result, the sum of the distances from the two foci to any point on the ellipse is 10. Since the two foci are on the major axis by definition, then the length of the major axis always represents the total distance from the two foci to any point on the ellipse.

The focus is interesting in our story of the planets as well. Recall Kepler identified the sun is at a focus of the ellipse that the planets travel on. The orbit of each planet creates a different ellipse, but the sun is always at a focus.

The ellipse and the circle

Now that we can visualize how an ellipse also illustrates the arithmetic and geometric means, let's view our circle and ellipse together to compare and contrast them. Since we centered our ellipse at the origin, we will also center our circle at the origin to make it easier to compare them. Of course, moving the circle in the $xy$-coordinate system does not change any of the properties we revealed in our field goal example.

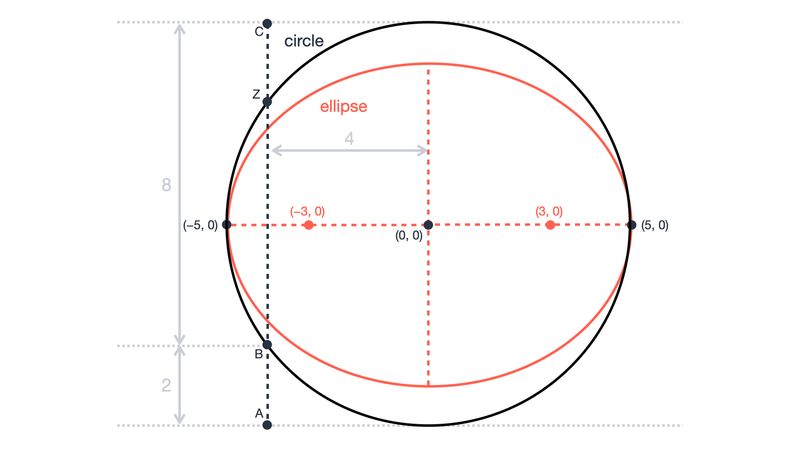
From this ellipse, we know the two numbers are 2 and 8 because the length of the major axis is 10 and the distance from a focus to the vertex is either 2 or 8. We also know the arithmetic mean is 5 because the distance from the center of the ellipse to a vertex is 5. Likewise, we know the geometric mean is 4 because the distance from the center of the ellipse along the minor axis to a point on the ellipse is 4.

The circle has a radius of 5, which indicates the arithmetic mean is 5. The geometric mean of the circle depends on where we draw a vertical line through the circle. Since we want a geometric mean of 4, travel 4 units to the left of the center. Then, draw a vertical line through that point and plot the points $A,B,Z,$ and $C$. We know from our field goal problem that the distance from $A$ to $B$ is 2 and the distance from $B$ to $C$ is 8. In summary, both the circle and ellipse illustrate the arithmetic and geometric means for the numbers 2 and 8.

There are other similarities between the ellipse and the circle. For example, the sum of the distance from the center of the circle to any two points on the circle is 10, while the sum of the distance from the two foci to any point on an ellipse is 10.

Visualizing the means

To be sure it’s clear how these two shapes display the two means, let’s view them on the same graph and focus only on the measurements that produce our two numbers (2 and 8) and our two means (4 and 5).

We’ll identify all our key values in three steps. Step 1: Identify our two key numbers. For the circle, the distance from $A$ to $B$ is 2 and the distance from $B$ to $C$ is 8. For the ellipse, the distance from $G$ to $H$ is 2 and the distance from $H$ to $E$ is 8.

Step 2: Calculate the arithmetic mean as the distance from $D$ to $E$, which is 5. For the circle, this is the radius. For the ellipse, this is the distance from the center of the ellipse to the vertex.

Step 3: Calculate the geometric mean, which is 4. For the circle, the geometric mean is the distance from $F$ to $D$. For the ellipse, it is the distance from $D$ to $I$. Remember we originally used our circle formula as a solution to a field goal problem. We presented the circle for the field goal problem a bit differently, but the concept was the same. Likewise, Kepler saw the connection between how planets orbit around the sun and music. In order for us to understand how Kepler connected music and an ellipse, we must change the ellipse size to one that highlights the math of music.

A musical ellipse

Recall the note one octave above the note $A_5$ is $A_6$. Also, recall we identified that $A_5$ has a frequency of 880 and $A_6$ has a frequency twice $A_5$’s frequency, which is 1,760. Let’s identify the arithmetic and geometric means of 880 and 1,760 by viewing an ellipse, and then we’ll identify how this ellipse connects to music. Recall the formula for an ellipse depends on the two means. First, let’s calculate the means. The arithmetic mean is $\frac{880+1760}{2}=1,320$ and the geometric mean is $\sqrt{880 \times 1,760}=1,244.51$. That gives us the ellipse with the equation $\frac{x^2}{1,320^2}$ + $\frac{y^2}{1,244.51^2} = 1$.

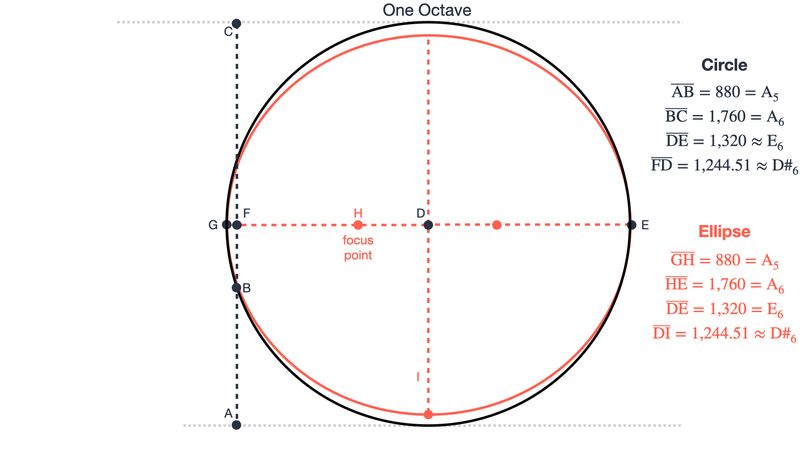
The remaining important points of the ellipse are the two foci. Since we know our two starting numbers are 880 and 1,760, we know the distance from one vertex, $G$, to the nearest focus, $H$, is 880 and then the distance from point $H$ to the other vertex, $E$, is 1,760.

Now that we have our ellipse drawn and identified, how can we connect this ellipse to music? Since the distance from point $G$ to $H$ is 880, we can view this distance as the frequency for $A_5$. Likewise, the distance from $H$ to $E$ is 1,760, which is the frequency for $A_6$.

Then, the distance from $D$ to $E$ is 1,320, which is approximately the frequency for $E_6$. Likewise, the distance from $D$ to $I$ is 1,244.51, which is approximately the frequency for $D\#_6$. Since the sun is at a focus, or point $H$, we can say the distance from the earth to the sun when the earth is closest to the sun, or point $G$, is like playing the note $A_5$. When the earth is farthest away from the sun, or point $E$, it is like playing the note $A_6$. Then, when the earth is halfway between these points, or point $I$, the distance to the center of our solar system, or point $D$, is like playing the note $E_6$.

If we have an imaginative perspective, we can say the solar system is playing a symphony for those with ears to hear.

A musical circle

Since we have been pairing a circle with an ellipse in this section, without much effort, let’s identify these notes on a circle. Recall the radius for the circle is the arithmetic mean, so draw a circle with radius 1,320. Using our previous notation, this is the distance from $D$ to $E$, which represents $E_6$. Then, identify the geometric mean by traveling 1,244.51 from the center of the circle directly to the left, which is from $D$ to $F$, or note $D\#_6$. Next, draw a vertical line that goes through this point. Notice this is our previous field goal problem. We can calculate the distance from the hash marks to each of the goal posts. The distance to the nearest goal post or the distance from $A$ to $B$ is 880, which is $A_5$. The distance from $B$ to $C$ is 1,760, which is $A_6$.

A circle and ellipse sing together

Once we understand how a circle and an ellipse relate to one octave, it is easy to repeat the formulas for two octaves. Consider the two octaves from $A_4$, which has a frequency of 440, to $A_6$, which has a frequency of 1,760. Now, our two numbers are 440 and 1,760. Here is a graph of the circle and ellipse that illustrates the music between these two octaves.

Notice the arithmetic mean is 1,100, which is approximately the note $C\#_6$. What do you think is the geometric mean? The geometric mean is 880, which is $A_5$, the perfect octave between $A_4$ and $A_6$. Observe the shape for the ellipse is flatter for the two-octave case compared to the one-octave case. This is because we increased the distance between our two numbers from one octave to two octaves, resulting in the geometric mean being much less than the arithmetic mean. There are additional insights to uncover by comparing a circle to an ellipse, but I will let you discover these interesting relationships on your own.